Question

If the sum of a number and seven is tripled, the result is eight times a number.

Answer 1

Step 1: Represent a with an unknown

Let a number be x

Step 2: Represent the given information with an equation

From the first statement, the sum of a number and seven is tripled can be represented with the equation below:

`(x+7)*3`

The second statement reflects that the result of the first statement is eight times a number. Eight times a number is

`8* x=8x`

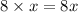
The result of the first statement and the second statement is

`(x+7)*3=8x`
`3x+21=8x`

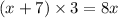
Step 3: Solve for the unknown

The solution of the unknown is as shown below:

`\begin{gathered} 3x+21=8x \\ \text{collect like-terms} \\ 21=8x-3x \\ 21=5x \\ \text{divide through by 5} \\ (21)/(5)=(5x)/(5) \\ (21)/(5)=x \end{gathered}`

Hence, the number is 4 1/5