Question

What is the max/min of the quadratic equation in factored form: f(x) = 0.5(x +3)(x-7)

Answer 1

F(x) = 1/2(x+3)(X-7)

Step 1 ; expand the function

F(x)= 1/2(x²-7x+3x-21)

F(x) = 1/2(x² - 4x-21)

F(x) = 1/2x² - 2x-21/2

Step 2 : Take the second derivative of F(x)

This means you are to differentiate F(X) twice

`\begin{gathered} F(x)=(1)/(2)x^2-2x-(21)/(2) \\ \text{First derivative is} \\ F^!(x)\text{=x-2} \\ F^(!!)(x)=1 \\ \text{the second derivative =1} \end{gathered}`

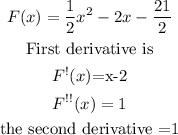
The second derivative is greater than 0, so it is a minimum point

Put x=1 in F(x) to find the value

`\begin{gathered} f(x)=(1)/(2)(1)^2_{}-\text{ 2(1)-}(21)/(2) \\ f(x)=(1)/(2)-2-(21)/(2) \\ f(x)=-2-(20)/(2) \\ f(x)\text{ =-12} \end{gathered}`

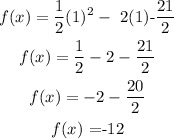
The minimum of the quadratic equation is -12