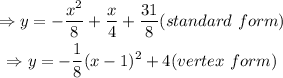Question

Write the equation for a parabola with a focus at (1,2) and a directrix at y=6

Answer 1

Given:

`\begin{gathered} focus=(1,2) \\ directrix,y=6 \end{gathered}`

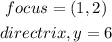
Step 1:

The equation of a parabola is given below as

`\begin{gathered} y=(1)/(4(f-k))(x-h)^2+k \\ (h,f)=focus \\ h=1,f=2 \end{gathered}`

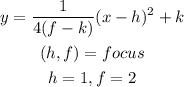
Step 2:

The distance from the focus to the vertex is equal to the distance from the vertex to the directrix:

`\begin{gathered} f-k=k-6 \\ 2-k=k-6 \\ 2k=2+6 \\ 2k=8 \\ (2k)/(2)=(8)/(2) \\ k=4 \end{gathered}`

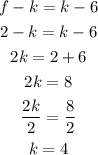
Step 3:

Substitute the values in the general equation of a parabola, we will have

`\begin{gathered} y=(1)/(4(f-k))(x-h)^(2)+k \\ y=(1)/(4(2-4))(x-1)^2+4 \\ y=-(1)/(8)(x-1)^2+4 \\ \end{gathered}`

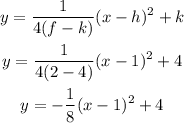
By expanding, we will have

`\begin{gathered} y=-(1)/(8)(x-1)^(2)+4 \\ y=-(1)/(8)(x-1)(x-1)+4 \\ y=-(1)/(8)(x^2-x-x+1)+4 \\ y=-(1)/(8)(x^2-2x+1)+4 \\ y=-(x^2)/(8)+(x)/(4)-(1)/(8)+4 \\ y=-(x^2)/(8)+(x)/(4)-(1+32)/(8) \\ y=-(x^2)/(8)+(x)/(4)+(31)/(8) \end{gathered}`

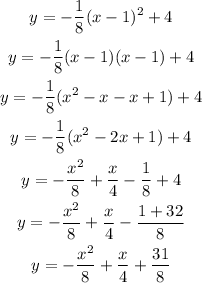
Hence,

The final answer is

`\begin{gathered} \Rightarrow y=-(x^(2))/(8)+(x)/(4)+(31)/(8)(standard\text{ }form) \\ \Rightarrow y=-(1)/(8)(x-1)^2+4(vertex\text{ }form) \end{gathered}`