Turn 5 7/10 into a decimal and a percent

Question

asked Dec 16, 2023 32.0k views

1 Answer

Phil Whittington · Answer 1 · 2023-12-22T17:41:11+0000

It is a mixed number:

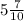
THe whole part, 5, is going to stay 5.

Now, let's work with the fractional part.

7/10 into a decimal would require for division.

7 divided by 10 is 0.7

Thus,

IN Decimal, it will be "5.7"

To find percentage from decimal, we multiply by 100 and tag along a % sign.

So,

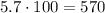
$5.7\cdot100=570$

The number, in percentage, is 570%