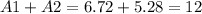Question

For each triangle identify a base and corresponding height use them to find the are

Answer 1

A)

For this tringle we can turn the figure like this:

now we have two right triangles and we can calulate the base of the first triangle with the sin law

`\begin{gathered} (\sin (90))/(3)=(sin(a))/(2.5) \\ \sin (a)=(2.5\sin (90))/(3) \\ \sin (a)=0.8 \\ a=\sin ^(-1)(0.8)=53º \end{gathered}`

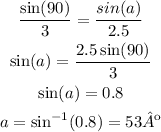
the angle b is going to be:

`\begin{gathered} 180=90+53+b \\ b=180-90-53 \\ b=37 \end{gathered}`

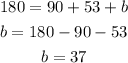
Now the base is going to be:

`\begin{gathered} (\sin(90))/(3)=\frac{\sin(37)}{\text{base}} \\ \text{base}=(3\sin (37))/(\sin (90))=1.8 \end{gathered}`

and the base of the secon triangle is going to be:

`\text{base}2=7.2-1.8=5.4`

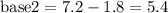
And the area of the triangles is going to be:

`A1=(base*2.5)/(2)=(1.8*2.5)/(2)=2.25`
`A2=(base2*2.5)/(2)=(5.4*2.5)/(2)=6.75`

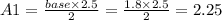
so in total the area is going to be:

`A1+A2=2.25+6.75=9`

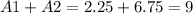
B)

the procedure is similar, first we turn the tiangle like this:

the angle a is going to be:

`\begin{gathered} \frac{\text{sin(a)}}{4.8\text{ }}=(\sin (90))/(6) \\ \sin (a)=(4.8\sin (90))/(6)=0.8 \\ a=\sin ^(-1)(0.8) \\ a=53º \end{gathered}`

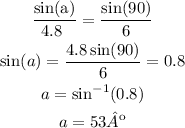
the angle b is going to be:

`\begin{gathered} 180=90+53+b \\ b=180-90-53 \\ b=37º \end{gathered}`

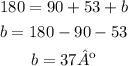
now the base is going to be:

`\begin{gathered} (\sin (37))/(base)=(sen(90))/(4.8) \\ \text{base}=(4.8\sin (37))/(\sin (90)) \\ \text{base}=2.8 \end{gathered}`

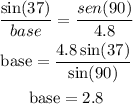
and the base of the other triangle will be:

`\text{base}2=5-2.8=2.2`

And the area of the triangles will be:

`\begin{gathered} A1=(base*4.8)/(2)=(2.8*4.8)/(2)=6.72 \\ A2=(base2*4.8)/(2)=(2.2*4.8)/(2)=5.28 \end{gathered}`

And the total area will be:

`A1+A2=6.72+5.28=12`