Answer:
0.135% or 0.00135
Explanation:
• The population mean height = 68 inches
,
• The population standard deviation = 4 inches
,
• Sample Size, n = 36
First, find the sample standard deviation:
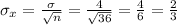
Next, for X=70, find the z-score:

Since we are looking for the probability that their average height is more than 70 inches, we need to find:
• P(X>70)=P(z>3)
Using the z-score table:
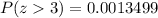
The probability that their average height is more than 70 inches is 0.135%.