Question

the variables x and y vary inversely. use x=-2 and y=3 to write and equation relating x and y. then find y when x=-1

Answer 1

Given the question in the question tab, the following are the solution steps to answer the question.

STEP 1: Define the variation that occurs in the Question.

Inverse Variation: Inverse variation is the relationship between two variables, such that if the value of one variable increases then the value of the other variable decreases.

STEP 2: Interpret the statements in the question tab

`\begin{gathered} x\text{ varies inversely as y} \\ x\propto(1)/(y) \end{gathered}`

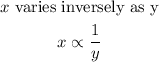
STEP 3: Get the constant of variation

`\begin{gathered} x\propto(1)/(y) \\ \text{Introducing the constant, we have;} \\ x=k*(1)/(y),x=(k)/(y) \\ By\text{ cross multiplication,} \\ x=ky \\ \text{Divide both sides by y} \\ (x)/(y)=k \end{gathered}`

STEP 4: Use the given values to get the equation relating x and y

`\begin{gathered} (x)/(y)=k,x=-2,y=3 \\ By\text{ substitution,} \\ (-2)/(3)=k \\ k=(-2)/(3) \\ \\ \text{The equation relating x and y will be:} \\ x=-(2)/(3)y \\ x=(-2y)/(3) \end{gathered}`

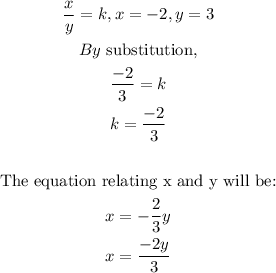
Hence, the equation relating x and y is:

`x=(-2y)/(3)`

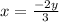
STEP 5: Find y when x=-1

`\begin{gathered} x=ky \\ \text{Divide both sides by k to get the value of y} \\ y=(x)/(k) \\ x=-1,k=-(2)/(3) \\ By\text{ substitution,} \\ y=(-1)/((-2)/(3)) \\ y=-1/-(2)/(3) \\ y=-1*(-3)/(2)=(-1*-3)/(2) \\ y=(3)/(2) \end{gathered}`

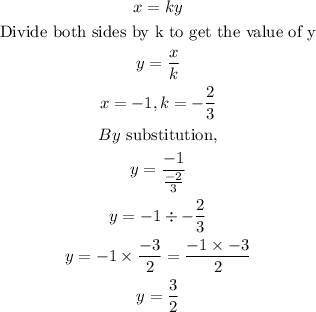
Hence, the value of y when x=-1 is 3/2