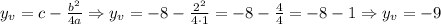Question

The Max or Min can be found by using the line of symmetry. That line of symmetry can be found by finding the midpoint of the two x-intercepts.Since the line of symmetry is x =-1 Write the function rule to find the coordinate to the minimum of this parabola.
`f (x) = (x - 2)(x + 4)`your answer should be in the form (_,_)

Answer 1

We know that, for a parabola, the minimum, or the maximum, is given by the vertex of the parabola. The formula for the vertex of the parabola is given by:

`x_v=-(b)/(2a),y_v=c-(b^2)/(4a)`

And we have the coordinates for x and y for the vertex.

We can see that the line of symmetry is x = -1, and this is the same value for the value of the vertex for x-coordinate, that is, the x-coordinate is equal to x = -1.

With this value for x, we can find the y-coordinate using the given equation of the parabola:

`f(x)=(x-2)\cdot(x+4)\Rightarrow f(-1)=(-1-2)\cdot(-1+4)\Rightarrow f(-1)=(-3)\cdot(3)`

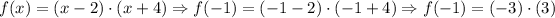
We can also expand these two factors, and we will get the same result:

`f(x)=(x-2)\cdot(x+4)=x^2+2x-8=(-1)^2+2\cdot(-1)-8=1-2-8=-1-8=-9`

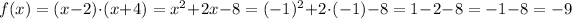
Therefore, the value for the y-coordinate (the value for the y-coordinate of the parabola, which is, at the same time, the minimum point for y of the parabola) is:

`f(-1)=(-3)\cdot(3)\Rightarrow f(-1)=-9`

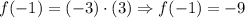
The minimum point of the parabola is (-1, -9) (answer), and we used the given function (rule) to find the value of the y-coordinate.

We can check these two values using the formula for the vertex of the parabola as follows:

`f(x)=(x-2)\cdot(x+4)=x^2+2x-8`

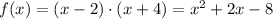
Then, a = 1 (it is positive so the parabola has a minimum), b = 2, and c = -8.

Hence, we have (for the value of the x-coordinate, which is, at the same time, the value for the axis of symmetry in this case):

`x_v=-(2)/(2\cdot1)\Rightarrow x_v=-1`

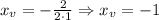
And for the value of the y-coordinate, we have:

`y_v=c-(b^2)/(4a)\Rightarrow y_v=-8-(2^2)/(4\cdot1)=-8-(4)/(4)=-8-1\Rightarrow y_v=-9`