The equation of circle is given by,
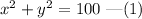
The equation of line is given by,
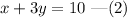
The points of intersection of the circle and line is,
A=(Xa, Ya)=(10, 0)
B=(Xb, Yb)=(-8, 6)
The length of chord AB can be calculated using distance formula as,
![\begin{gathered} AB=\sqrt[]{(X_b-X_a)^2+(Y_b-Y_a)^2} \\ =\sqrt[]{(-8-10)^2+(6-0)^2} \\ =\sqrt[]{(-18)^2+6^2} \\ =\sqrt[]{324+36} \\ =\sqrt[]{360} \\ =6\sqrt[]{10} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/jxsgpf0m78q33ealokradj5fv910dq78jm.png)
Let (Xc, Yc) be the coordinates of point C on the circle. Hence, using equation (1), we can write
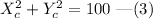
Using distance formula, the expression for the length of chord AC is given by,
![AC=\sqrt[]{(X_c^{}-X_a)^2+(Y_c-Y_a)^2_{}}](https://img.qammunity.org/2023/formulas/mathematics/college/5b54c5xn0w8sf6t2d2xapcm8u2fei03bjt.png)
Since (Xa, Ya)=(10, 0),
![\begin{gathered} AC=\sqrt[]{(X^{}_c-10_{})^2+(Y_c-0_{})^2_{}} \\ AC=\sqrt[]{(X^{}_c-10_{})^2+Y^2_c} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/ddzdyznxi84fjufjo1qxl200evza9bin3v.png)
It is given that chords AB and AC have equal length. Hence, we can write
![\begin{gathered} AB=AC \\ 6\sqrt[]{10}=\sqrt[]{(X^{}_c-10_{})^2+Y^2_c} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/9uw4koolpj98yw6rlrenhg5cnjxxhzftmx.png)
Squaring both sides of above equation,
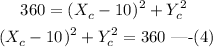
Subtract equation (4) from (3) and solve for Xc.

Put Xc=-8 in equation (3) to find Yc.
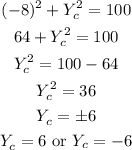
So, the coordinates of point C can be (Xc, Yc)=(-8, 6) or (Xc, Yc)=(-8, -6).
Since (-8, 6) are the coordinates of point B, the coordinates of point C can be chosen as (-8, -6).
Therefore, the coordinates of point C is (-8, -6) if chords AB and AC have equal length.