Question

4. McKenzie wants to determine which ice cream option is the best choice. The chart below gives the description and prices for her options. Use the space below each item to record your findings. Place work below the chart. A scoop of ice cream is considered a perfect sphere and has a 2-inch diameter. A cone has a 2-inch diameter and a height of 4.5 inches. A cup, considered a right circular cylinder, has a 3-inch diameter and a height of 2 inches. a. Determine the volume of each choice. Use 3.14 to approximate pi. b. Determine which choice is the best value for her money. Explain your reasoning. (That means some division, you decide which.) $2.00 $3.00 $4.00 One scoop in a сир Two scoops in a cup Three scoops in a cup Half a scoop on a cone filled with ice cream A cup filled with ice cream (level to the top of the cup)

Answer 1

McKenzie wants to determine which ice cream option is the best choice.

Part (a)

Volume of Scoop:

A scoop of ice cream is considered a perfect sphere and has a 2-inch diameter.

The volume of the sphere is given by

`V=(4)/(3)\cdot\pi\cdot r^3`

Where r is the radius.

We know that radius is half of the diameter.

`r=(D)/(2)=(2)/(2)=1`

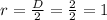
So, the volume of a scoop of ice cream is

`V_{\text{scoop}}=(4)/(3)\cdot3.14\cdot(1)^3=(4)/(3)\cdot3.14\cdot1=4.19\: in^3`

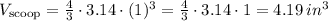
Therefore, the volume of a scoop of ice cream is 4.19 in³

Volume of Cone:

A cone has a 2-inch diameter and a height of 4.5 inches.

The volume of a cone is given by

`V=(1)/(3)\cdot\pi\cdot r^2\cdot h`

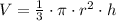
Where r is the radius and h is the height of the cone.

We know that radius is half of the diameter.

`r=(D)/(2)=(2)/(2)=1`

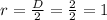
So, the volume of a cone of ice cream is

`V_{\text{cone}}=(1)/(3)\cdot3.14\cdot(1)^2\cdot4.5=(1)/(3)\cdot3.14\cdot1^{}\cdot4.5=4.71\: in^3`

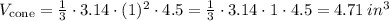
Therefore, the volume of a cone of ice cream is 4.71 in³

Volume of Cup:

A cup, considered a right circular cylinder, has a 3-inch diameter and a height of 2 inches.

The volume of a right circular cylinder is given by

`V=\pi\cdot r^2\cdot h`

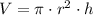
Where r is the radius and h is the height of the right circular cylinder.

We know that radius is half of the diameter.

`r=(D)/(2)=(3)/(2)=1.5`

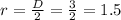
So, the volume of a cup of ice cream is

`V_{\text{cup}}=3.14\cdot(1.5)^2\cdot2=3.14\cdot2.25\cdot2=14.13\: in^3`

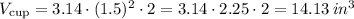
Therefore, the volume of a cup of ice cream is 14.13 in³

Part (b)

Now let us compare the various given options and decide which option is the best value for money

Option 1:

The price of one scoop in a cup is $2

The volume of one scoop of ice cream is 4.19 in³

`rate=(4.19)/(\$2)=2.095\:`

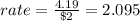
Option 2:

The price of two scoops in a cup is $3

The volume of one scoop of ice cream is 4.19 in³

`rate=(2\cdot4.19)/(\$3)=2.793\:`

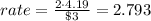
Option 3:

The price of three scoops in a cup is $4

The volume of one scoop of ice cream is 4.19 in³

`rate=(3\cdot4.19)/(\$4)=3.1425`

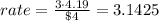
Option 4:

The price of half a scoop in a cone is $2

The volume of one scoop of ice cream is 4.19 in³

The volume of one cone of ice cream is 4.71 in³

`rate=((4.19)/(2)+4.71)/(\$2)=(2.095+4.71)/(\$2)=(6.805)/(\$2)=3.4025`

Option 5:

The price of a cup filled with ice cream is $4

The volume of a cup is 14.13 in³

`rate=(14.13)/(\$4)=3.5325`

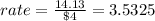
As you can see, the option 5 (a cup filled with ice cream) has the highest rate (volume/$)

This means that option 5 provides the best value for money.

Therefore, McKenzie should choose "a cup filled with ice cream level to the top of cup" for the best value for money.