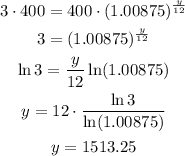Question

Leah invested $400 in an account paying an interest rate of 1 1/2%compounded annually. Lauren invested $400 in an account paying aninterest rate of 0 7/8% compounded monthly. To the nearest hundredth of ayear, how much longer would it take for Lauren's money to triple than forLeah's money to triple?

Answer 1

Leah investment is:

`M_{\text{Leah}}=400_{}\cdot1.5^y`

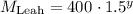
Where M is the ammount of money that she has, and y the number of years.

We want to know the number of years that must elapse for her investment to triple, so we want to know the value of y such that:

`\begin{gathered} 3\cdot400=400\cdot(1+(1.5)/(100))^y \\ 3=(1.015)^y \\ \ln 3=y\cdot\ln (1.015) \\ y=(\ln (3))/(\ln (1.015))\cong73.788\cong73.79 \end{gathered}`

It will take 73.79 years to triple her investment.

Lauren investment is:

`M_{\text{Lauren}}=400\cdot(1+(7)/(8)\cdot(1)/(100))^m=400\cdot(1.00875)^{(y)/(12)}`

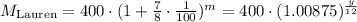
Where M is the ammount of money that she has, and m the number of months, and y is the number of years.

We want to know the number of years that must elapse for her investment to triple, so we want to know the value of y such that:

`\begin{gathered} 3\cdot400=400\cdot(1.00875)^{(y)/(12)} \\ 3=(1.00875)^{(y)/(12)} \\ \ln 3=(y)/(12)\ln (1.00875) \\ y=12\cdot(\ln 3)/(\ln (1.00875)) \\ y=1513.25 \end{gathered}`