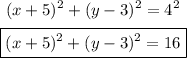Question

Determine the equation of the graphed circleReminder that the equation should look like the example I provided

Answer 1

The equation of a circle of radius r and center at (h, k) is:

`(x-h)^2+(y-k)^2=r^2`

The image provided shows a circle and we must find the radius and center by simple inspection.

The center is located at (-5, 3).

From that point, until I find a point of the circumference I can count 4 units. It is confirmed when I see the segment from (-9, 3) to (-1, 3) as a diameter of length 8. The radius is half the diameter, thus r = 4.

Substituting, we have the required equation:

`\begin{gathered} (x+5)^2+(y-3)^2=4^2 \\ \boxed{\mleft(x+5\mright)^2+\mleft(y-3\mright)^2=16} \end{gathered}`