Question

Draw a reference triangle and find the trig ratio for the angle (if possible) without a calculator.

Answer 1

Recall the definition of the cosecant function in terms of the sine function:

`\csc (x)=(1)/(\sin (x))`

Draw the angle -210º in the unit circle to find a reference triangle for that angle:

Notice that a 30-60-90 triangle is formed:

Since the side opposite to the 30º angle has a length of 1/2, then:

`\sin (30º)=(1)/(2)`

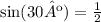
On the other hand, notice that since the angle of -210º lies in the second quadrant, then the value of sin(-210º) is positive:

`\sin (-210º)=\sin (30º)=(1)/(2)`

Use the definition of the cosecant function to find csc(-210º):

`\csc (-210)=(1)/(\sin (-210º))=(1)/(((1)/(2)))=2`

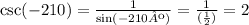
Therefore, the value of csc(-210º) is equal to 2.