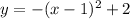Question

Writing the equation of a quadratic function given its graph

Answer 1

`y=-(x-1)^2+2`

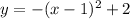
Explanation:

A quadratic function in vertex form is represented as:

`\begin{gathered} y=a(x-h)^2+k \\ \text{where,} \\ (h,k)\text{ is the vertex} \end{gathered}`

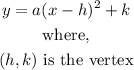
Given the vertex (1,2) substitute it into the function:

`y=a(x-1)^2+2`

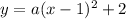
As you can see, we still do not know the value for ''a'', use the point given (4,-7) substitute it (x,y) and solve for ''a'':

`\begin{gathered} -7=a(4-1)^2+2 \\ -7=a(3)^2+2 \\ -7=9a+2 \\ 9a=-7-2 \\ a=-(9)/(9) \\ a=-1 \end{gathered}`

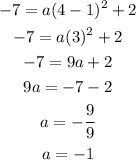
Hence, the equation of the function would be:

`y=-(x-1)^2+2`