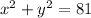The equation of the circle has the following form:

Where
(h,k) are the coordinates of the center of the circle
r is the radius of the circle
If the center of the circle is at the origin, (0,0) and it passes through the point (0,-9), since both x-coordinates are equal, the length of the radius is equal to the difference between the y-coordinates of the center and the given point:
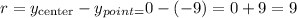
The radius is 9 units long.
Replace the coordinates of the center and the length of the radius in the formula:

So, the equation of the circle that has a center in the origin and passes through the point (0.-9) is: