Question

Suppose you deposit $600 into an account that pays 5% annual interest, compounded continuously. How much will you have in the account in 4 years? ƒ(t) = ae^rt

Answer 1

To determine the amount that will be on the account after 4 years you have to apply the given exponential function that models the amount of money on the account with respect to the time.

`f(t)=ae^(rt)`

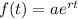
Where

a represents the initial amount

r represents the interest rate expressed as a decimal value

t is the time period in years

The initial amount on the account is a= $600

The time period is t= 4 years

The interest rate is r=5%, divide it by 100 to express it as a decimal value:

`r=(5)/(100)=0.05`

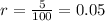
Using this information, you can calculate the final amount:

`\begin{gathered} f(t)=ae^(rt) \\ f(4)=600e^(0.05\cdot4) \\ f(4)=600e^(0.2) \\ f(4)=732.84 \end{gathered}`

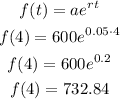
After 4 years there will be $732.84 on the account. The correct option is B.