Answer: Choice C)
0.3821, 0.8745
========================================================
Work Shown:
pi/2 = 3.14/2 = 1.57 approximately
The solutions for t must be in the interval 0 ≤ t ≤ 1.57

where n is an integer.
Let
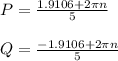
Then let's generate a small table of values like so
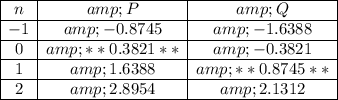
The terms with surrounding double stars represent items in the interval 0 ≤ t ≤ 1.57
Therefore, we end up with the solutions 0.3821 and 0.8745 both of which are approximate.
You can use a graphing tool like Desmos or GeoGebra to verify the solutions. Be sure to restrict the domain to 0 ≤ t ≤ 1.57