Question

15

4. If M is the midpoint of line segment EF. E is located at (10,-8) and M is located at (-6, -2). Find the other
endpoint.

Answer 1

F = (-22, 4)

Explanation:

Midpoint between two points

`\textsf{Midpoint}=\left((x_2+x_1)/(2),(y_2+y_1)/(2)\right)\\\\ \textsf{where $(x_1,y_1)$ and $(x_2,y_2)$ are the endpoints.}`

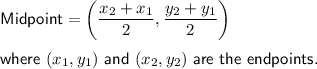
Given information:

• Midpoint = (-6, -2)
• E = (10, -8)

Define the endpoints of the line segment EF:

• Let (x₁, y₁) = endpoint E = (10, -8)
• Let (x₂, y₂) = endpoint F

Substitute the given information into the formula:

`\implies (x_M,y_M)=\left((x_F+x_E)/(2),(y_F+y_E)/(2)\right)`

`\implies (-6,-2)=\left((x_F+10)/(2),(y_F-8)/(2)\right)`

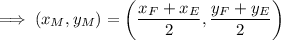
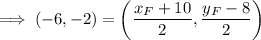
Find the x-coordinate of M:

`\implies (x_F+10)/(2)=-6`

`\implies x_F+10=-12`

`\implies x_F=-22`

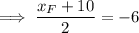
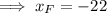
Find the y-coordinate of M:

`\implies (y_F-8)/(2)=-2`

`\implies y_F-8=-4`

`\implies y_F=4`

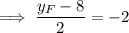
Therefore, the coordinates of endpoint F are (-22, 4).

Answer 2

• The other endpoint is F( - 22, 4)

=============================

Given

Segment EF with:

• Endpoint E = (10, - 8),
• Midpoint M = (- 6, - 2),
• Endpoint F = (x, y).

Solution

Use midpoint equation:

• x = (x₁ + x₂)/2, y = (y₁ + y₂)/2

Find unknown coordinates of the point F:

• - 6 = (10 + x)/2 ⇒ -12 = 10 + x ⇒ x = - 12 - 10 = - 22,
• - 2 = (- 8 + y)/2 ⇒ - 4 = - 8 + y ⇒ y = - 4 + 8 = 4.

So the point is F = ( - 22, 4)