Answer:
∠DHG = 72°
Explanation:
As ∠DHG and ∠EHF are vertical angles, they are congruent.
What are vertical angles?
Vertical angles are formed when two lines intersect, creating opposite angles. Those opposites are congruent (equal).
Equation:
∠DHG = ∠EHF
[6(x - 2)] = (3x + 30)
Step 1: Distribute 6 through the parentheses.
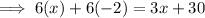
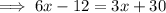
Step 2: Subtract 3x from both sides.
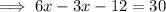

Step 3: Add 12 to both sides.
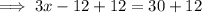
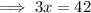
Step 4: Divide both sides by 3.

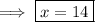
Step 5: Substitute the value of x into ∠DHG to find its measure.
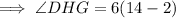
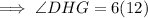
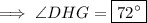
Therefore, the measure of ∠DHG is 72°.