Question

16. A rectangular picture measures 6 inches by 8 inches. Simon wants to build a

wooden frame for the picture so that the framed picture takes up a maximum area
of 100 square inches on his wall. The pieces of wood that he uses to build the
frame all have the same width. Write an equation or inequality that could be used
to determine the maximum width of the pieces of wood for the frame Simon could
create.
Explain how your equation or inequality models the situation.
Solve the equation or inequality to determine the maximum width of the pieces of
wood used for the frame to the nearest tenth of an inch.

Answer 1

`w \leq 1.9`

Explanation:

The picture itself measures 6" by 8". Therefore, the area is 6 * 8 = 48 square inches.

All of the pieces of wood have the same width, and we know that two of them will be 6 inches long and two will be 8 inches long.

Therefore, the combined area of the pieces of wood will be 2 * 6w and 2 * 8w. This gives us 12w + 16w.

Now we can set up an inequality:

48 (area of picture) + 12w + 16w <= 100 (maximum area).

Simplify the left side of the equation to give us this:

`48+28w\leq 100.`

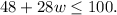
We can move the 48 over to the other side to get
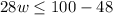`28w \leq 100 - 48`, which simplifies to
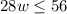`28w \leq 56`. Now, you just have to divide both sides by 28 to get
`w\leq 1.857`.

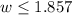Rounding to the nearest tenth of an inch gives us 1.9 as the maximum width of the pieces of wood.