Answer:
3619.1 grams (nearest tenth)
Explanation:
Volume of a cylinder
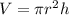
where:
- r is the radius.
- h is the height.
The radius of a circle is half its diameter.
Find the volumes of the external and internal cylinders by substituting the given values into the formula for volume of a cylinder:
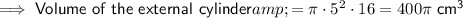

Find the volume of the cylindrical hollow metal pipe by subtracting the volume of the internal cylinder from the volume of the external cylinder:
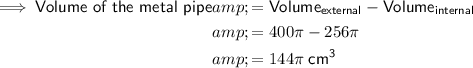
Density formula

where:
- ρ = density
- m = mass
- V = volume
Given:
Substitute the given values of density and the found volume into the density formula and solve for m:

Therefore, the mass of the pipe is 3619.1 grams (nearest tenth).
Note: I have used the exact value of π (not a rounded value).