Question

Write an equation for the intervals of a parabola with x-intercepts at (3,0) and (9,0) that passes through the point (10,-7).

Help is always greatly appreciated.

Answer 1

`\quad \huge \quad \quad \boxed{ \tt \:Answer }`

`\qquad\displaystyle \tt \rightarrow \: y= - {x}^(2) +12x - 27`

____________________________________

`\large \tt Solution \: :`

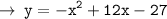
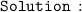
The values of x where a parabola cuts the x - axis (y = 0) are the roots of the quadratic equation.

I.e 3 and 9 for the given problem.

and the equation can be represented as :

`\qquad\displaystyle \tt \rightarrow \: y = a(x - x1)(x- x2)`

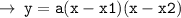
where, x1 and x2 are the roots of the quadratic equation, a is a constant value (depicting strech in curve)

Now, plug in the values :

`\qquad\displaystyle \tt \rightarrow \: y= a(x- 3)(x - 9)`

`\qquad\displaystyle \tt \rightarrow \: y = a( {x}^(2) -9x - 3x +27)`

`\qquad\displaystyle \tt \rightarrow \: y= a( {x}^(2) -12 +27)`

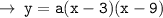
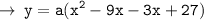
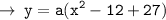
Now, we need to find the value of a, for that let's use the coordinates of a point lying on the curve (10 , -7)

`\qquad\displaystyle \tt \rightarrow \: - 7= a( {10}^(2) -12(10) +27)`

`\qquad\displaystyle \tt \rightarrow \: - 7= a(100- 120+27)`

`\qquad\displaystyle \tt \rightarrow \: - 7= a( 7)`

`\qquad\displaystyle \tt \rightarrow \: a = ( - 7) / ( 7)`

`\qquad\displaystyle \tt \rightarrow \: a = -1`

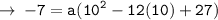
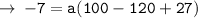
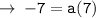
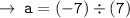
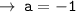
Now, we got all required values. let's plug the value of a in equation, and we will get the required equation of parabola.

`\qquad\displaystyle \tt \rightarrow \: y= -( {x}^(2) -12x +27)`

`\qquad\displaystyle \tt \rightarrow \: y= - {x}^(2) +12x - 27`

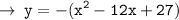
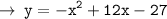
Answered by : ❝ AǫᴜᴀWɪᴢ ❞

Answer 2

`y=-x^2+12x-27`

Explanation:

Intercept form of a quadratic equation

`\boxed{y=a(x-p)(x-q)}`

where:

• p and q are the x-intercepts.
• a is some constant.

Given x-intercepts:

• (3, 0)
• (9, 0)

Substitute the given x-intercepts into the formula:

`\implies y=a(x-3)(x-9)`

To find a, substitute the given point (10, -7) into the equation and solve for a:

`\implies a(10-3)(10-9)=-7`

`\implies a(7)(1)=-7`

`\implies 7a=-7`

`\implies a=-1`

Therefore, the equation of the function in factored form is:

`\implies y=-(x-3)(x-9)`

Expand the brackets:

`\implies y=-(x^2-9x-3x+27)`

`\implies y=-(x^2-12x+27)`

`\implies y=-x^2+12x-27`

Therefore, the equation of the function in standard form is:

`\boxed{y=-x^2+12x-27}`