Question

Determine the more basic function that has been shifted reflected stretched or compressed what does

Answer 1

`f(x)=√(x)`

Step-by-step explanation

The graph of a function f is the set of all points in the plane of the form (x, f(x)).

Step 1

iven

`g(x)=-2√(x-1)+4`

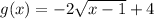
g(x) is a transformation of the function f(x)we have a root in the function, note that the variable x is inside the root, so we can conclude the basic function is

square tooroot

Step 2

rove

ow, lets's check the transformation of f(x)

`f(x)=√(x)`

a)1 was subtracted form the argument of the fucnction

`\begin{gathered} f(x)=√(x) \\ f^(\prime)(x)=√(x-1) \end{gathered}`

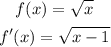
when you subtract a number b form the argument of the function you are shifting the function b units to the rigth

so

he function was shifteed 1 unit to rigth

) the resulting function was multiplied by 2-

`\begin{gathered} f^(\prime)(x)=√(x-1)\text{ *-2} \\ f^(\prime)^(\prime)(x)=-2√(x-1) \end{gathered}`

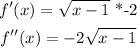
when you multplie by a negavitve constant you are reflecting the funciton acrros x-axis, and is is stretched vertically.

so

he funcition was reflected across x-axis an strtched vertically by a factor of 2

c) 4 was added to the function

`\begin{gathered} f^(\prime\prime)(x)=-2√(x-1) \\ g(x)=-2√(x-1)+4 \end{gathered}`

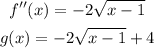
when you add a constant b to any function, the graph will be shifted b units up,so

he functinon was shifted 4 units up

hrerefore, we can conclude the answer is

`f(x)=√(x)`

I hope this helps you