Question

The length of AB to the nearest hundredth

Answer 1

Answer: 7.28

Explanation:

This answer is solved by using the Pythagorean Theorem in a clever way. As we know, the Pythagorean Theorem states that:
`a^2 + b^2 = c^2`

Rewriting this, we find out that it:
`c = √(a^2 + b^2)`

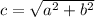
We are using the value C to be the length of AB.

Now that we know how we are solving for c, we can now find values for a and b.

What we want to do, is find sides that will complete a triangle with AB. These sides are measures as:
`x_2 - x_1` (where
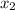`x_2` is the x value of coordinate B, and
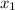`x_1` is the x-value of coordinate A) and
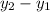`y_2 - y_1` (where y is similar to x).

Now that we have our sides, we can plug them in to the theorem we rewrote before:

`c = √((x_2 - x_1)^2 + (y_2 - y_1)^2) = √((-3 - (-5))^2 + (3 - (-4))^2)`

We can then begin to solve for AB:

`AB = √((2)^2 + (7)^2) = √(4 + 49) = √(53) = 7.28`

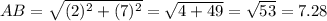
I hope this helps. If you need anything explained more please let me know.

Answer 2

8

Explanation:

you count the blocks starting from a to b