Question

The foot of a ladder is placed 7 meters from a wall if The top of the ladder rests 9 meters up on the wall how long is the ladder

Answer 1

11.40

Explanation:

in a right angle triangle

the distance from the wall is the adjacent

the height of the wall is the opposite.

using Pythagoras theorem, hypotenuse²= opposite²+adjacent²

hypotenuse²= 7²+9²=49+81=130

hypotenuse=✓130=11.4

Answer 2

11.4018 meters

Explanation:

The ladder, wall and floor together form a right triangle with the length of the ladder being the hypotenuse, the distance of the foot of the ladder from the wall being one of the shorter sides and the distance between the top of the ladder and the floor being the other side

We can use the Pythagorean theorem to solve for the hypotenuse given the two sides

The general formula is:

`c = \sqrt{a^(2) + b^(2)}`

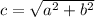
where c is the hypotenuse and a and b the other two sides

Substituting a = 7 and b = 9 gives:

`c = \sqrt{a^(2) + b^(2)}\\\\c = \sqrt{7^(2) + 9^(2)}\\\\c = √(49 + 81)\\\\c = √(130)\\\\c = 11.4018`

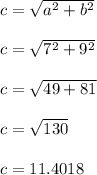
So length of ladder = 11.4018 meters