Answer:
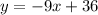
Explanation:
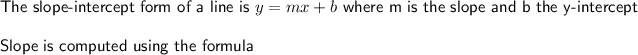
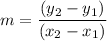
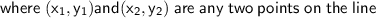


So the slope of the line is y = -9x + b
We have
y = -9x + b
Add 9x to both sides
y + 9x = -9x + 9x + b
y + 9x = b
switching sides,
b = y + 9x
Plug in the (x, y) values for point (4, 0)
b = 0 + 9(4)
b = 36
So the equation of the line is
