Question

Igcse math (quadratic functions)

given the equation: x^2 + 5x - 14
use the discriminant to decide if there are two real and different roots, two equal roots or no real roots

Answer 1

2 real different roots

Explanation:

Discriminant determines the number of real solutions of a quadratic equation. The formula of discriminant goes by:

`\displaystyle{D = b^2-4ac}`

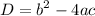
The formula is derived from a quadratic formula which is:

`\displaystyle{x=(-b\pm √(b^2-4ac))/(2a)}`

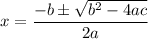
The expression inside the square root is discriminant. The discriminant says that:

• There are 2 real different roots if the discriminant (D) is greater than 0. (D > 0)
• There is 2 real double roots (same roots) if the discriminant (D) is equal to 0. (D = 0)
• There are no real roots (imaginary or complex roots) if the discriminant (D) is less than 0. (D < 0)

From the equation
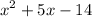`\displaystyle{x^2+5x-14}`, determine the coefficients of equation:

• a = 1
• b = 5
• c = -14

Therefore, substitute the coefficients’ values in the discriminant:

`\displaystyle{D=5^2-4(1)(-14)}\\\\\displaystyle{D=25-4(-14)}\\\\\displaystyle{D=25+56}\\\\\displaystyle{D=81}`

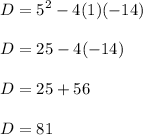
Since the discriminant is greater than 0, we can conclude that this equation will have 2 real different roots.