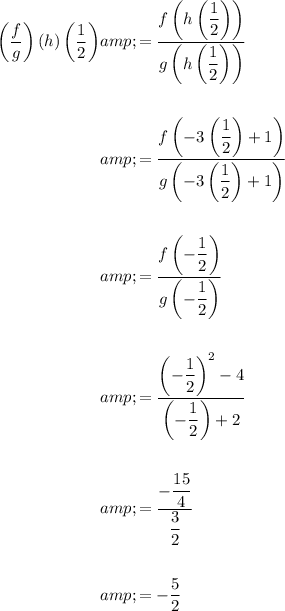Answer:
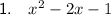


Explanation:
Given functions:
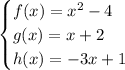
Question 1
The composite function (f + g - h)(x) means to add functions f(x) and g(x) then subtract function h(x):
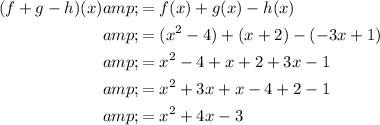
Question 2
The composite function (fgh)(x) means to multiply functions f(x), g(x) and h(x):

Question 3
The composite function (f/g)(h)(1/2) means to substitute the value of function h(x) when x = 1/2 into function f(x) and function g(x) and divide the former by the latter: