Question

If f(a) = 3a – a2, which of the following are not true statements? Select all that apply. f(-5) = -40 f(4) = -4 f(-1) = 2 f(0) = 3 f(3) = 0

Answer 1

Key Ideas

• Solving with functions

Solving the Question

We're given:

• 
  `f(a) = 3a - a^2`

Determine f(-5):

`f(a) = 3a - a^2\\f(-5) = 3(-5) - (-5)^2\\f(-5) = -15 - 25\\f(-5) = -40`

Therefore, this statement is true.

Determine f(4):

`f(a) = 3a - a^2\\f(4) = 3(4) - (4)^2\\f(4) = 12 - 16\\f(4) = -4`

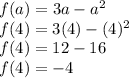
Therefore, this statement is true as well.

Determine f(-1):

`f(a) = 3a - a^2\\f(-1) = 3(-1) - (-1)^2\\f(-1) = -3 - 1\\f(-1) = -4`

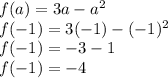
Therefore, this statement is not true.

Determine f(0):

`f(a) = 3a - a^2\\f(0) = 3(0) - (0)^2\\f(0) = 0 - 0\\f(0)=0`

Therefore, this statement is also not true.

Answer

f(-5) = -40

f(4) = -4