Question

Write the equation of a quadratic with the vertex at (2,-3) and passing through the point (6,4)

Answer 1

`\displaystyle\\Answer:\ y=(7)/(16)x^2 -(7)/(4)x-(5)/(4)`

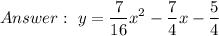
Explanation:

The vertex is also the symmetry point of the parabola. The formula for finding the x-coordinate of the parabola: x = -b/2a (2,-3)

Hence,

`\displaystyle\\2=(-b)/(2a) \\`

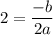
Multiply both parts of the equation by -2a:

`\displaystyle\\-4a=b\ \ \ \ \ (1)`

`y=ax^2+bx+c\ \ \ \ \ -\ \ \ \ \ the\ quadratic\ equation\\\\Thus,`

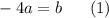
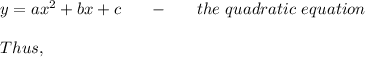
You can make a system of equations on two points belonging to the quadratic equation:

`-3=a(2)^2+b(2)+c\\4=a(6)^2+b(6)+c\\\\-3=4a+2b+c\ \ \ \ (2)\\4=36a+6b+c\ \ \ \ (3)\\\\`

Substitute (1) into equations (2) and (3):

`-3=4a+2(-4a)+c\\4=36a+6(-4a)+c\\\\-3=4a-8a+c\\4=36a-24a+c\\\\-3=-4a+c\ \ \ \ (4)\\4=12a+c \ \ \ \ (5)\\\\\\`

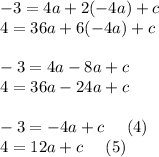
Subtract equation (4) from equation (5):

`7=16a`

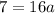
Divide both parts of the equation by 16:

`\displaystyle\\(7)/(16) =a\ \ \ \ (6)`

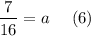
Substitute (6) into equations (1):

`\displaystyle\\-4((7)/(16) )=b\\\\-(4*7)/(4*4)=b\\\\-(7)/(4)=b`

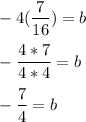
Substitute values a and b into equation (2):

`\displaystyle-3=4((7)/(16))+2(-(7)/(4))+c\\\\ -3=(7)/(4) -(14)/(4)+c\\\\ -3=-(7)/(4)+c \\\\-3+(7)/(4)=-(7)/(4)+c+(7)/(4) \\\\ (-3*4+7)/(4) =c\\\\(-12+7)/(4)=c\\\\ -(5)/(4)=c`

Thus,

`\displaystyle\\y=(7)/(16)x^2 -(7)/(4)x-(5)/(4)`