Question

Slope of (-8,-3) and (-1,-2)

Answer 1

We have that the data are:

(x₁ = -8, y₁ = -3) and (x₂ = -1, y₂ = -2)

The slope is equal to the change in and with respect to change in X, or what rises when moving forward.

`\boxed{\large\displaystyle\text{$\begin{gathered}\sf \bf{m=(Change \ in \ y)/(Change \ in \ x) \ \longmapsto \ \ m=(y_(2)-y_(1))/(x_(2)-x_(1)) } \end{gathered}$}}`

The change in X is equal to the subtraction in the X coordinate (also called advance), and the change in and is equal to the subtraction in the coordinate and (also called elevation).

Introduce the values of x and y In the equation to find the slope.

`\boxed{\large\displaystyle\text{$\begin{gathered}\sf \bf{m=(-2-(-3))/(-1-(-8) ) } \end{gathered}$}}`

`\boxed{\boxed{\large\displaystyle\text{$\begin{gathered}\sf \bf{m=(1)/(7) } \end{gathered}$}}}`

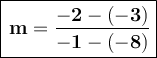
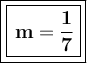
The slope of (-8, -3) and (-1, -2) is 1/7.

Answer 2

Solution :-

As we know that,

• m = y_2 - y_1 / x_2 - x_1

Therefore ,

>> m = -2 - (-3) / -1 - (-8)

>> m = -2 + 3 / -1 + 8

>> m = 1 / 7