Question

On a number line, point A is at coordinate -2. Point B is at coordinate 0. Fine the coordinate that is the midpoint from A to B.

Answer 1

The midpoint of the line segment AB

is at (−1, 0).

Explanation:

Midpoint Formula:

In a general sense the midpoint formula for a line segment whose endpoints are

`\sf ( x_1 + x_2) (y_1 + y_2)`

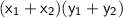
would be the mean of the abscissa and the ordinate as seen in the formula below:

`\sf \: (x_m, \: y_m) = ( (x_1 + x_2)/(2) , (y_1 + y_2)/(2) )`

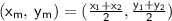
When the line segment lies on one axis, then the there would be only one non-zero value in the ordered pair.

Assuming that the line segment AB

is in the x-axis, then the coordinates of the points would be:

`\sf \: A(−2, 0)B(0,0)`

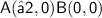
To get the midpoint, substitute the points above in the formula for the midpoint:

`\sf \: (x_m, \: y_m) = ( (x_1 + x_2)/(2) , (y_1 + y_2)/(2) ) \\ \sf \: = ( ( - 2 + 0)/(2) ),0 \\ \sf \: = ( ( - 2)/(2) ), 0 \: \: \: \: \: \: \: \: \\ \sf \: ( - 1, 0)`

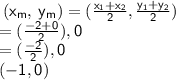
The midpoint of the line segment AB

is at (−1, 0).