Answer:
y = {-1/4 for x < -3; +1/4 for x > -3}
see attached for a graph
Explanation:
You want the function y = |x+3|/(4x+12) written as a piecewise function and graphed.
Absolute value function
The absolute value function resolves into two pieces, each with its own domain. These let you write the function as a piecewise function.
(x+3) < 0
When (x+3) < 0, the absolute value function negates this value:
y = -(x+3)/(4(x+3)) = -1/4 . . . . . for x < -3
(x+3) ≥ 0
When (x+3) ≥ 0, the absolute value function does nothing. That means the equation becomes ...
y = (x +3)/(4(x +3))
When the denominator is zero, the function is undefined. When the denominator is not zero, the function becomes ...
y = 1/4 . . . . . for x > -3
Piecewise function
Then the definition of the piecewise function is ...
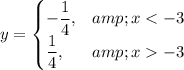
The graph is attached.