Question

Given the function f(x)=8, find and simplify the difference quotient.

Please include your math and/or explain! I can figure out all the other ones with difference quotient, but this one is tripping me up. Thank you very much!

Answer 1

Answer: 0

=================================================

Work Shown:

f(x) = 8

f(x+h) = 8

Compute the difference quotient

`(f(x+h) - f(x))/(h) = (8 - 8)/(h)\\\\(f(x+h) - f(x))/(h) = (0)/(h)\\\\(f(x+h) - f(x))/(h) = 0 \ \text{ where } h \\e 0\\\\`

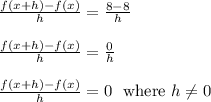
Notice that the function y = 8 is a horizontal line through 8 on the y axis. The slope of the tangent is the exact same as the linear function, so this tangent slope is also 0. This is why we end up with a difference quotient of 0.

Another thing to note is that f(x) and f(x+h) are identical simply because no x is found in the f(x) function. The output is always 8.