Question

Find the inverse of f(x)=2x-1

Answer 1

`(x+1)/(2)`

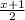
Explanation:

The inverse of a function
`f(x)` is denoted by f
`^(-1)(x)`

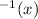
To find the inverse of
`f(x) = 2x -1`

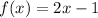
Set
`y = f(x)`

So

`y = 2x-1`

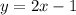
Express x in terms of y

`y+1 = 2x \textrm{ or } 2x = y + 1`

`x=(y+1)/(2)`

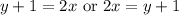
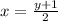
So inverse is
`f^(-1)(x) =`
`(x+1)/(2)`

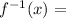
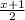
Replace y with x to make it a function of x

`f^(-1)(x) =`
`(x+1)/(2)`

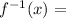
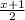
To test if this correct, note that the property of an inverse function is that it undoes the original function. In other words if we have f(a) = k then f⁻¹(k) = a

Let's test this with some reasonable value for x

f(2) = 2.2 - 1 = 3

f⁻¹(3) = (3+1)/2 = 2

Hence correct

Answer 2

`{ \qquad\qquad\huge\underline{{\sf Answer}}}`

Let's find the inverse of given function ~

`\qquad \sf  \dashrightarrow \: f(x) = 2x - 1`

`\qquad \sf  \dashrightarrow \: 2x = f(x) + 1`

`\qquad \sf  \dashrightarrow \: x = \cfrac{f(x) + 1}{2}`

[ now, replace f(x) and x with
`\sf{ {f}^(-1)(x)}` ]

`\qquad \sf  \dashrightarrow \: f {}^( - 1) (x) = \cfrac{x + 1}{2}`

That's the required inverse function ~