Question

Solve these 4 equations. Tell me if they have one, none, or many solutions.

1. 3(x+4)=3x+11
2. -2(x+3)=-2x-6
3. 4(x-1) = 1/2(x-8)
4. 3x-7=4+6 +4x

Answer 1

1. 3(x+4)=3x+11: No solutions.

2. -2(x+3)=-2x-6: Infinite solutions, any number can make the statement true.

3. 4(x-1) = 1/2(x-8): x= 0.

4. 3x-7=4+6 +4x: x= -17.

Explanation:

Equation 1.

1. Write the expression.

`3(x+4)=3x+11`

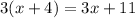
2. Simplify the left side of the equation by applying the associative property of multiplication.

This is the rule we're applying:
`a(b+c)=(a*b)+(a*c)`.

`(3)(x)+(3)(4)=3x+11\\ \\3x+12=3x+11`

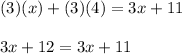
3. Substract 3x from both sides.

`3x+12-3x=3x+11-3x\\ \\12=11`

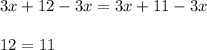
4. Conclude.

As you may notice, we just obtained a false statement in subtitle 3, because 12 doesn't equal 11. Hence, this equation doesn't have solutions.

-------------------------------------------------------------------------------------------------------

Equation 2.

1. Write the expression.

`-2(x+3)=-2x-6`

2. Simplify the left side of the equation by applying the associative property of multiplication.

`(-2)(x)+(-2)(3)=-2x-6\\ \\-2x-6=-2x-6`

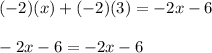
3. Conclude.

Whenever we get an equation that has the same arguments on both sides, this means that there are infinite solutions to this equation, any value of x will make the equation true. Hence, the equation has infinite solutions.

-------------------------------------------------------------------------------------------------------

Equation 3.

1. Write the expression.

`4(x-1) = 1/2(x-8)`

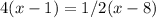
2. Simplify the both sides of the equation by applying the associative property of multiplication.

`(4)(x)+(4)(-1) = ((1)/(2) )(x)+((1)/(2) )(-8)\\ \\4x-4=(1)/(2) x-4`

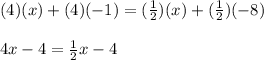
3. Add 4 to both sides.

`4x-4+4=(1)/(2) x-4+4\\ \\4x=(1)/(2) x`

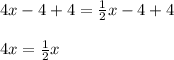
4. Substract
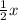`(1)/(2) x` from both sides.

`4x-(1)/(2) x=(1)/(2) x-(1)/(2) x\\ \\4x-(1)/(2) x=0\\ \\4x-0.5x=0\\ \\3.5x=0\\ \\x=(0)/(3.5 ) \\ \\x=0`

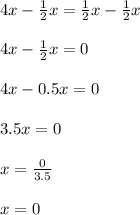
5. Concluide.

The solution for this equation is x= 0.

-------------------------------------------------------------------------------------------------------

Equation 4.

1. Write the expression.

`3x-7=4+6 +4x`

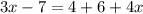
2. Simplify by completing the addition.

`3x-7=10 +4x`

3. Add 7 to both sides.

`3x-7+7=10 +4x+7\\ \\3x=17+4x`

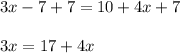
4. Substract 4x from both sides.

`3x-4x=17+4x-4x\\ \\-x=17`

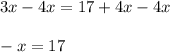
5. Multiply bith sides by -1.

`(-1)(-x)=(17)(-1)\\ \\x=-17`

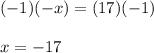
6. Conclude.

The solution of this equation is x= -17.

Answer 2

1. no solutions

2. many (infinite) solutions

3. one solution: x = 0

4. one solution: x = -17

Explanation:

Question 1

`\begin{aligned}&\textsf{Given equation}: & 3(x+4)&=3x+11\\&\textsf{Distribute}: & 3x+12&=3x+11\\&\textsf{Subtract }3x \textsf{ from both sides}: & 12 &=11\end{aligned}`

As 12 ≠ 11 there are no solutions.

Question 2

`\begin{aligned}&\textsf{Given equation}: & -2(x+3)&=-2x-6\\&\textsf{Distribute}: & -2x-6 &=-2x-6\\&\textsf{Add }2x \textsf{ to both sides}: & -6 &=-6\\&\textsf{Add }6 \textsf{ to both sides}: & 0 & = 0\\\end{aligned}`

As 0 = 0 there are infinite (many) solutions.

Question 3

`\begin{aligned}&\textsf{Given equation}: & 4(x-1) & =(1)/(2)(x-8)\\&\textsf{Distribute}: & 4x-4 &=(1)/(2)x-4\\&\textsf{Add }4 \textsf{ to both sides}: & 4x & = (1)/(2)x\\&\textsf{Subtract } (1)/(2)x \textsf{ from both sides}: & (3)/(2)x & = 0\\&\textsf{Divide both sides by } (3)/(2): & x & = 0\end{aligned}`

Therefore, there is one solution, x = 0.

Question 4

`\begin{aligned}&\textsf{Given equation}: & 3x-7 & = 4+6+4x\\&\textsf{Simplify}: & 3x-7 & = 10+4x\\&\textsf{Swap sides}: & 10+4x & = 3x-7\\&\textsf{Subtract 10 from both sides}: & 4x & = 3x-17\\&\textsf{Subtract }3x \textsf{ from both sides}: & x & = -17\end{aligned}`

Therefore, there is one solution, x = -17.