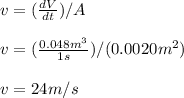Question

120 litres of water is discharge from container in 25 seconds. Find the rate of discharge in cumecs.if the discharge took place from pipe of diameter 50 mm. find the velocity flow​

Answer 1

Rate of discharge in cumecs: 0.0048m³/s.

Velocity flow: 24m/s.

Step-by-step explanation:

1. Find the rate of discharge in cumecs.

a. Convert from litres to m³.
120L*1000= 120000mL

120000mL=120000cm³

120000cm³/100³=0.12 m³.

b. Rate of discharge.

If 0.12 m³ where discharged in 25 seconds, the rate of discharge is:

0.12m³/25s = 0.0048m³/s.

2. Find the velocity flow.

Let's refer to the fluid mechanics equation that relates volume flow, area and velocity. This is the formula:

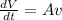`(dV)/(dt)=Av`; where the expression
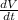`(dV)/(dt)` is the volume flow rate (in m³/s); A is the cross-sectional area of the pipe (in m²), and v is the velocity flow (in m/s).

a. Solve the equation for v.

`(dV)/(dt)=Av\\ \\((dV)/(dt))/A=v\\ \\v=((dV)/(dt))/A`

b. Calculate the cross-sectional area of the pipe.

The cross-sectional area of the pipe is a circle. Hence, the formula of this area is:

`A=\pi r^(2)`

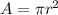
We'll have to convert the diameter to meters, because the formula for flow velocity needs the area in m². Let's go ahead and do that.

`50mm/1000=0.05m`.

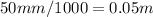We were given the diameter, and the formula uses the radius, but the radius is just half of the diameter, therefore, we can substitute in toe formula like this:

`A=\pi ((0.05)/(2) )^(2)=0.0020m^(2)`

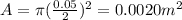
c. Substitute in the new expression for velocity flow and calculate.

`v=((dV)/(dt))/A\\ \\v=((0.048m^(3) )/(1s))/(0.0020m^(2) )\\\\ v= 24m/s`