Question

What is the slope of a line perpendicular to the line whose equation is 15x-12y=216. Fully simplify your answer.

Answer 1

`-(4)/(5)`.

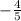Explanation:

1. Find the slope of the given equation.

To find this value, we need to solve the equation for y.

`15x-12y=216\\\\-12y=-15x+216\\\\y=(-15x)/(-12) +(216)/(-12)\\\\ y=(5x)/(4) -18`

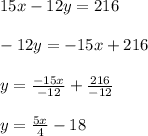
The slope of a linear equation is always the number by which the x in being multiplied when the equation is solved for y. Hence, the value of the slope is
`(5)/(4)` .

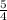2. Find slope of the original line.

The problem gives us information to find the slope of the line perpendicular to
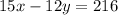`15x-12y=216`. Since the slope of the given equation is
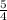`(5)/(4)` , the slope of a perpendicular line is the multiplicative reciprocal with the opposite sign. This is inverting the fraction, and adding the opposite symbol of what the original fraction had, like this:

a. We invert the fraction.

We previously had
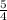`(5)/(4)`, now we'll have
`(4)/(5)`

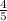
b. Add the opposite sign before the fraction.

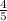`(4)/(5)` doesn't have a sign before it, that means that it is positive. Converting it to negative we have
`-(4)/(5)`.

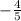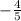`-(4)/(5)` is our final answer.