∑ Hey, zayzaygaming2009 ⊃
Answer:
D. x= -74 and x = 80
Explanation:
Given:
Solve for x: 1/7 (x - 3) - 2 = 9
A. x = 74 and x = 80
B. x = -46 and x =52
C. x = -52 and x = 46
D. x= -74 and x = 80
Solve:
I think you meant:
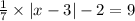 because if you solve
because if you solve
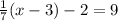 the answer will be x= 80 but as I look at the answer choices it doesn't match.
the answer will be x= 80 but as I look at the answer choices it doesn't match.
This is a absolute value equation.
To solve this absolute value equation we have to isolate the absolute value part first. Which is;
⇒
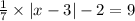
⇒
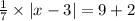
⇒
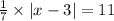
⇒
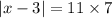
⇒
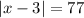
Instantly, we can decompose the absolute value equation into two simpler ones below:
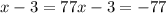
Now we solve for x;
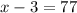
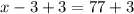

and;
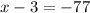
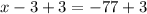
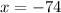
Hence the solutions are;
x = -74 and x = 80
Which also means:
D. x = -74 and x = 80 is the correct answer.
xcookiex12
8/18/2022