Answer:
ΔU= U2-U1= 7.35 J - 0 J= 7.35 J.
Step-by-step explanation:
Point A= 0 m.
Point B= 0.25 m.
Gravitational potential energy formula:
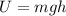 , where m is the mass (in kg) of the body. G is the magnitude of the gravitational field that the body is being exposed to. H is the height at which the body is located at.
, where m is the mass (in kg) of the body. G is the magnitude of the gravitational field that the body is being exposed to. H is the height at which the body is located at.
1. First, let's calculate gravitational potential energy of the car on point A:
U₁= (3.0 kg)(9.8 m/s²)(0 m)= 0 J.
2. Gravitational potential energy of the car on point B:
U₂= (3.0 kg)(9.8 m/s²)(0.25 m)= 7.35 J.
Now, calculating the change in the car’s gravitational potential energy:
ΔU= U₂-U₁= 7.35 J - 0 J= 7.35 J.