Question

A bucket of paint has spilled on a tile floor. The paint flow can be expressed with the function p(t) = 6t, where t represents time in minutes and p represents how far the paint is spreading.

The flowing paint is creating a circular pattern on the tile. The area of the pattern can be expressed as A(p) = πp2.

Part A: Find the area of the circle of spilled paint as a function of time, or A[p(t)]. Show your work.

Part B: How large is the area of spilled paint after 8 minutes? You may use 3.14 to approximate π in this problem.

Answer 1

A(p(t))

• π(6t)²
• π36t²
• 36t²π

#B

put t=8

A(p(t))

• 36π(8)²
• 36π(64)
• 7234.56units²

Answer 2

A) A[p(t)] = 36πt²

B) 7234.56 square units

Explanation:

Given functions:

`\begin{cases}p(t)=6t \\ A(p)=\pi p^2 \end{cases}`

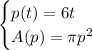
Part A

To find the area of the circle of spilled paint as a function of time, substitute the function p(t) into the given function A(p):

`\begin{aligned}A(p) & = \pi p^2\\\\ \implies A[p(t)] & = \pi [p(t)]^2\\& = \pi (6t)^2\\& = \pi 6^2 t^2\\& = 36\pi t^2\end{aligned}`

Part B

Given:

• t = 8 minutes
• π = 3.14

Substitute the given values into the equation for A[p(t)] found in part A:

`\begin{aligned}A[p(8)] & = 36\pi t^2\\& = 36 \cdot 3.14 \cdot 8^2\\& = 36 \cdot 3.14 \cdot 64\\& = 113.04 \cdot 64\\& = 7234.56\:\: \sf square\:units\end{aligned}`

Therefore, the area of spilled paint after 8 minutes is 7234.56 square units.