Question

How many committees of 4 boys and 3 girls

can be formed from a class of 6 boys and 7
girls?

Answer 1

525

Explanation:

This is a question involving combinatorics

The number of ways of choosing a subset k from a set of n elements is given by
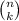`{n \choose k}` which evaluates to
`(n!)/(k!(n-k)!)`

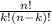
n! is the product n × (n-1) × (n-2) x....x 3 x 2 x 1

For example,

4! = 4 x 3 x 2 x 1 = 24

3! = 3 x 2 x 1 = 6

Since we have to choose 4 boys from a class of 6 boys, the total number of ways this can be done is

`{6 \choose 4} = (6!)/(4!(6-4)!) = (6!)/(4!2!)`

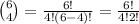
Note that 6! = 6 x 5 x 4 x 3 x 2 x 1 and 4 x 3 x 2 x 1 is nothing but 4!

So the numerator can be re-written as 6 x 5 x (4!)

We can rewrite the expression
`(6!)/(4!2!) \text{ as } (6.5.4!)/(4!2!)`

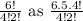
Cancelling 4! from both numerator and denominator gives us the result

as (6 × 5)/2! = 20/2 = 15 different ways of choosing 4 boys from a class of 6 boys

For the girls, the number of ways of choosing 3 girls from a class of 7 girls is given by

`{7 \choose 3} = (7!)/(3!(7-3)!) = (7!)/(3!4!)`

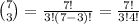
This works out to (7 x 6 x 5 )/(3 x 2 x 1) (using the same logic as for the boys computation)

= 210/6 = 35

So total number of committees of 4 boys and 3 girls that can be formed from a class of 6 boys and 7 girls = 15 x 35 = 525