Question

Find the area of the roof of the gazebo in problem 25, if each roof ridge is 10ft long, as shown

Answer 1

Check the picture below.

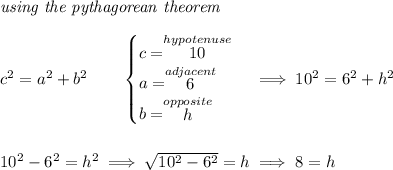
first off let's check what "h" is in the triangular face.

`\textit{using the pythagorean theorem} \\\\ c^2=a^2+b^2 \qquad \begin{cases} c=\stackrel{hypotenuse}{10}\\ a=\stackrel{adjacent}{6}\\ b=\stackrel{opposite}{h}\\ \end{cases}\implies 10^2=6^2+h^2 \\\\\\ 10^2-6^2=h^2\implies √(10^2-6^2)=h\implies 8=h`

so, the gazebo has 4 squarish faces, each 12x12, recall 48 ÷ 4 = 12, and it has 4 triangular faces, each with a height of 8 and a base of 12.

notice we're skipping the top and bottom of the cube and the bottom of the pyramid because they're not part of the "surface area".

`\stackrel{\textit{\large Areas}}{\stackrel{\textit{4 triangular faces}}{4\left[\cfrac{1}{2}(12)(8) \right]}~~~~+~~~~\stackrel{\textit{4 squarish faces}}{4[12\cdot 12]}}\implies 192+576\implies 768`