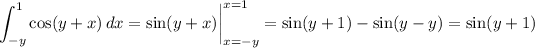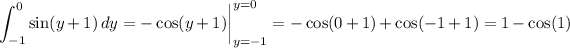Question

Unsure how to do this calculus, the book isn't explaining it well. Thanks

Answer 1

One way to capture the domain of integration is with the set

`D = \left\{(x,y) \mid 0 \le x \le 1 \text{ and } -x \le y \le 0\right\}`

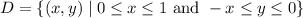
Then we can write the double integral as the iterated integral

`\displaystyle \iint_D \cos(y+x) \, dA = \int_0^1 \int_(-x)^0 \cos(y+x) \, dy \, dx`

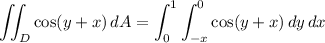
Compute the integral with respect to
`y`.

`\displaystyle \int_(-x)^0 \cos(y+x) \, dy = \sin(y+x)\bigg|_(y=-x)^(y=0) = \sin(0+x) - \sin(-x+x) = \sin(x)`

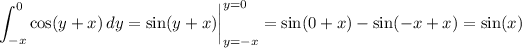
Compute the remaining integral.

`\displaystyle \int_0^1 \sin(x) \, dx = -\cos(x) \bigg|_(x=0)^(x=1) = -\cos(1) + \cos(0) = \boxed{1 - \cos(1)}`

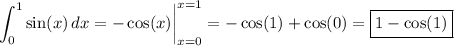
We could also swap the order of integration variables by writing

`D = \left\{(x,y) \mid -1 \le y \le 0 \text{ and } -y \le x \le 1\right\}`

and

`\displaystyle \iint_D \cos(y+x) \, dA = \int_(-1)^0 \int_(-y)^1 \cos(y+x) \, dx\, dy`

and this would have led to the same result.

`\displaystyle \int_(-y)^1 \cos(y+x) \, dx = \sin(y+x)\bigg|_(x=-y)^(x=1) = \sin(y+1) - \sin(y-y) = \sin(y+1)`

`\displaystyle \int_(-1)^0 \sin(y+1) \, dy = -\cos(y+1)\bigg|_(y=-1)^(y=0) = -\cos(0+1) + \cos(-1+1) = 1 - \cos(1)`