Answer:
Exponential function
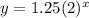
Explanation:
Definitions
Asymptote: a line that the curve gets infinitely close to, but never touches.
Hole: a point on the graph where the function is not defined.
Polynomial Function
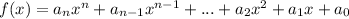
An equation containing variables with non-negative integer powers and coefficients, that involves only the operations of addition, subtraction and multiplication.
A continuous function with no holes or asymptotes.
Rational Function
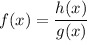
An equation containing at least one fraction whose numerator and denominator are polynomials.
A rational function has holes and/or asymptotes.
- A rational function has holes where any input value causes both the numerator and denominator of the function to be equal to zero.
- A rational function has vertical asymptotes where the denominator approaches zero.
- If the degree of the numerator is smaller than the degree of the denominator, there will be a horizontal asymptote at y = 0.
- If the degree of the numerator is the same as the degree of the denominator, there will be a horizontal asymptote at y = ratio of leading coefficients.
- If the degree of the numerator is exactly one more than the degree of the denominator, slant asymptotes will occur.
Logarithmic Function
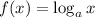
A continuous function with a vertical asymptote.
A logarithmic function has a gradual growth or decay.
Exponential Function
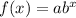
The variable is the exponent.
A continuous function with a horizontal asymptote.
An exponential function has a fast growth or decay.