Question

Please help!!
maths functions

Answer 1

The Co-ordinate of C is (4/3, -1/2)

Explanation:

We have two equation one is of straight line equation which is:

y=2x-3 (i)

Other equation is of quadratic function which is:

y=-3x^2+5 (ii)

Put the value of y from equation (i) in equation (ii)

So, we have:

2x-3=-3x^2+5

3x^2+2x-8=0

By factorization:

3x^2+6x-4x-8=0

3x(x+2)-4x(x+2)=0

(x+2)(3x-4)=0

x+2=0 ; 3x-4=0

x=-2 ; x=4/3

Put first x=-2 in equation (i)

y=2(-2)-3

y=-4-3

y=-7

Now Put x=4/3 in equation (i)

y=2(4/3)-3

y=8/3-3

y=-1/2

So, we have two Order pair One is (-2 , -7) and Second one is (4/3 , -1/2)

Hence the Co-ordinate of C is:

C=(4/3 , -1/2)

Answer 2

Point C: (3, 3)

Point D: (3, -22)

Explanation:

If the distance between points C and D is 25 units, the y-value of point D will be 25 less than the y-value of point C. The x-values of the two points are the same.

Therefore:

`\textsf{Equation 1}: \quad y=2x-3`

`\textsf{Equation 2}: \quad y-25=-3x^2+5`

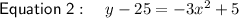
As the x-values are the same, substitute the first equation into the second equation and solve for x to find the x-value of points C and D:

`\implies 2x-3-25=-3x^2+5`

`\implies 3x^2+2x-33=0`

`\implies 3x^2-9x+11x-33=0`

`\implies 3x(x-3)+11(x-3)=0`

`\implies (x-3)(3x+11)=0`

`\implies x=3, -(11)/(3)`

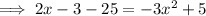
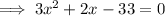
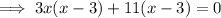
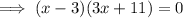
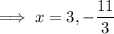
From inspection of the given graph, the x-value of points C and D is positive, therefore x = 3.

To find the y-value of points C and D, substitute the found value of x into the two original equations of the lines:

`\begin{aligned} \textsf{Point C}: \quad 2x-3 & =y\\2(3)-3 & =3\\ \implies & (3, 3)\end{aligned}`

`\begin{aligned} \textsf{Point D}: \quad -3x^2+5 & = y \\ -3(3)^2+5 & =-22\\ \implies & (3, -22)\end{aligned}`

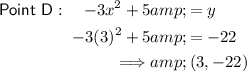
Therefore, point C is (3, 3) and point D is (3, -22).