Question

a writer wrote 10,010 words for his book on the first day of writing. he wrote 9,760 words on the second day, 9,510 words on the third day, and continued this way in an arithmetic sequence. write an explicit rule showing the equation for the number of words the writer would write on the 14th day, and solve.

Answer 1

• rule: an = 10010 -250(n -1)
• day 14: 6760 words

Explanation:

The first three terms of the arithmetic sequence for the number of words written are 10010, 9760, 9510. These have a common difference of 9760-10010 = -250. The first term and common difference can be used to make the explicit equation for the words written on the n-th day.

Formula for Arithmetic Sequence

The explicit formula for the n-th term of an arithmetic sequence with first term a₁ and common difference d is ...

`a_n=a_1+d(n-1)`

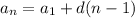
Application

For first term a₁ = 10010 and common difference d = -250, the explicit rule is ...

`a_n=10010-250(n-1)`

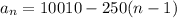
On day 14, n=14, and the number of words written is ...

`a_(14)=10010-250(14-1)\\\\a_(14)=10010-250(13)=10010-3250\\\\a_(14)=6760`

The writer would write 6760 words on the 14th day.