Answer:
The 99% two-sided confidence interval for p, the proportion of bearings with surface finish rougher than allowed specification is (0.1430, 0.3788). The upper bound of this 2-sided confidence interval is 0.3788.
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.

In which
z is the zscore that has a pvalue of
 .
.
In a random sample of 92 automobile engine crankshaft bearings, 24 have a surface finish that is rougher than the specifications allow.
This means that
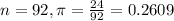
99% confidence level
So
 , z is the value of Z that has a pvalue of
, z is the value of Z that has a pvalue of
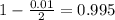 , so
, so
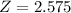 .
.
The lower limit of this interval is:
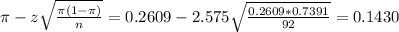
The upper limit of this interval is:
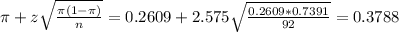
The 99% two-sided confidence interval for p, the proportion of bearings with surface finish rougher than allowed specification is (0.1430, 0.3788). The upper bound of this 2-sided confidence interval is 0.3788.