Question

The Rocky Mountain district sales manager of Rath Publishing Inc., a college textbook publishing company, claims that the sales representatives make an average of 39 sales calls per week on professors. Several reps say that this estimate is too low. To investigate, a random sample of 25 sales representatives reveals that the mean number of calls made last week was 40. The standard deviation of the sample is 3.8 calls. Using the 0.100 significance level, can we conclude that the mean number of calls per salesperson per week is more than 39?

H0:μ≤39
H1:μ>39
What is the decision regarding H0?

Answer 1

Following are the solution to these choices:

Explanation:

`\mu_(0)=39\\\\n = 25\\\\s = 3.8\\\\\bar{X}=40\\\\\alpha =0.1`

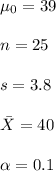
The test assumption is:

`\text{Null Hypothesis} \to H_0:\mu =\mu_(0)\\\\\text{Alternate Hypothesis} \to H_1:\mu >\mu_(0)\\\\`

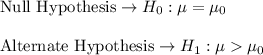
It is a checked right-tail, since the alternative hypothesis is produced to classify the argument when the data difference is greater than 0.

`\text{Critical value} =t_(\alpha,n-1) = t_(0.1, 24) =1.3178\\\\\text{Rejection Region:} t_(0) > t_(\alpha,n-1)\\\\\text{Since} \ t_(0) = 1.3158 < 1.3178 = t_(0.1)\\\\`

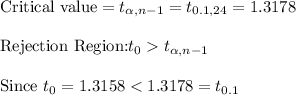
The null hypothesis should be rejected:
`H_(o): \mu = 39.0\ at\ \alpha =0.1.`

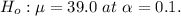
They have little enough proof that perhaps the average number of calls per person per week amounts to even more than 39.