Question

Steam enters a turbine operating at steady state with a specific enthalpy of 1407.6 Btu/lb and expands to the turbine exit where the specific enthalpy is 1236.4 Btu/lb. The mass flow rate is 5 lb/s. During this process, heat transfer to the surroundings occurs at a rate of 40 Btu/s. Neglecting kinetic and potential energy effects, the power developed by the turbine is

Answer 1

The power developed by the turbine is 816 BTU per second.

Step-by-step explanation:

Thermodynamically speaking, a turbine produces work at the expense of fluid energy. By First Law of Thermodynamics, the energy balance of a turbine working at steady state is:

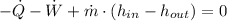`-\dot Q -\dot W +\dot m\cdot (h_(in)-h_(out)) = 0` (1)

Where:

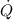`\dot Q` - Heat transfer rate, measured in BTU per second.

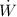`\dot W` - Power developed by the turbine, measured in BTU per second.

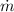`\dot m` - Mass flow rate, measured in pounds per second.

`h_(in)`,
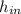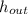`h_(out)` - Specific enthalpies at inlet and outlet, measured in BTU per pound.

If we know that
`\dot Q = 40\,(BTU)/(s)`,
`\dot m = 5\,(lbm)/(s)`,
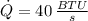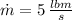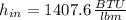`h_(in) = 1407.6\,(BTU)/(lbm)` and
`h_(out) = 1236.4\,(BTU)/(lbm)`, then the power developed by the turbine is:

`\dot W = -\dot Q + \dot m \cdot (h_(in)-h_(out))`

`\dot W = 816\,(BTU)/(s)`

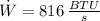
The power developed by the turbine is 816 BTU per second.